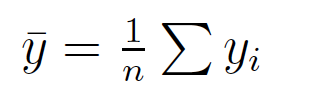Is it consistent? How can we derive whether an estimator of the parameter is consistent or not?
1 Answer
The definition of "consistent" is that the estimator approaches the truth as the sample size grows large. The parameter of interest is presumably $\mu_y$, the population expected value of $y$.
In this case, you just need to make assumptions on $y_i$ to determine if there is consistency.
If $\mu_y$ exists (not all distributions have a finite mean) and your data are independent and identically distributed, then by Kolmogorov's second strong law of large numbers,
$$\frac{1}{n}\sum_{i=1}^ny_i \rightarrow^{a.s.} \mu_y $$
In English, the estimator is consistent. The superscript "a.s." denotes almost sure convergence.
-
$\begingroup$ Thank you for answering all of my questions! $\endgroup$– SeraCommented Oct 30, 2022 at 19:20