Three types of Neutral technological Change

Question

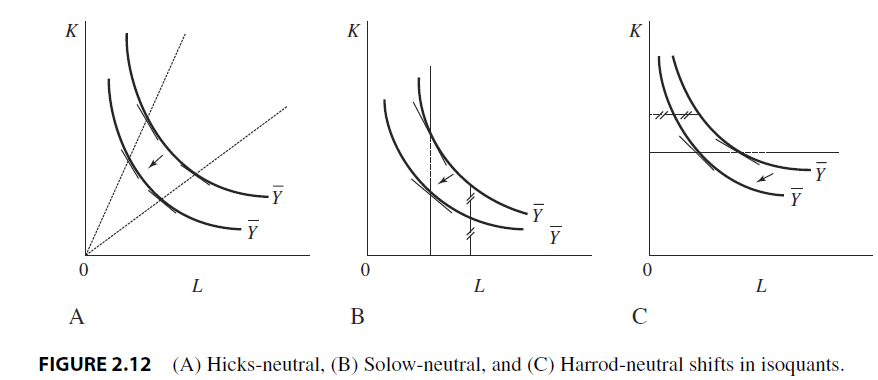
Can you tell me why the isoquants changes as per pic below in the three cases of neutral technological change: i) Hicks neutral, ii) Solow neutral, iii) Harrod neutral

Answer 1

Let us assume the Cobb-Douglas production function:

$Y = AK^{\alpha}L^{1-\alpha}$, here A is called total factor productivity (TFP)

$Y = K^{\alpha}(AL)^{1-\alpha}$, here A is labor augmenting technological change or Harrod-neutral technological change

$Y = (KA)^{\alpha}L^{1-\alpha}$, here A is capital augmenting technological change or Hicks-neutral technological change. Now let us interpret the graphs given:

Hicks-neutral technological change (Figure A):

• In Hicks-neutral technological change, the technology improvement affects the production process such that the ratio of capital to labor used in production remains constant for a given level of output.
• The isoquants shift inward uniformly. This means that the same level of output $\bar{Y}$ can now be produced with less of both capital $K$ and labor $L$, maintaining the same capital-to-labor ratio.
• This type of change reflects a proportional increase in productivity that affects all production processes equally without favoring the use of more capital or labor.

Solow-neutral technological change (Figure B):

• Solow-neutral (or capital-neutral) technological change refers to improvements where the efficiency of capital is increased, but the way labor is used does not change. This is often modeled as an increase in the effectiveness of capital alone.
• The isoquants in this case shift in a way that for a given level of output, less capital is needed, but the labor input remains relatively constant at higher output levels.
• The result is that capital becomes more productive, and thus less of it is required to achieve the same output, keeping labor input steady.

Harrod-neutral technological change (Figure C):

• Harrod-neutral (or labor-neutral) technological change improves the productivity of labor while leaving the productivity of capital unchanged.
• This leads to a decrease in the amount of labor needed to produce the same level of output while the capital usage per level of output does not change significantly.
• The isoquants shift such that less labor is required across all levels of output, but the usage of capital per unit of output stays relatively stable.