Taxation shifting the supply curve left, the demand left curve, why not both?

Question

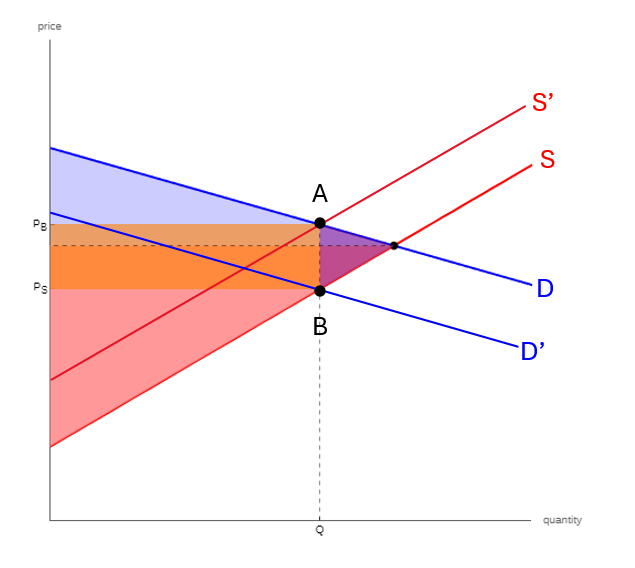
When we model supply and demand, I can understand why when we introduce the conception of taxation, we can model it as a shift to the left of the supply curve, this is because the marginal cost of production increases; so firms want to charge more at any given quantity to make up for the tax, so to speak.

I can also understand why it could be a shift to the left in demand, say I usually buy 3 apples for \$12 each, now there is a \$2 tax; that's going to reduce the amount I want to spend (not including tax) to \$10, it's going to reduce my willingness to pay.

The issue I have is when we just say "well it can be modelled by one or the other," because the result (that the price demanders pay is the same amount higher than the price suppliers pay, i.e. P_s + tax = P_d) is going to be true in either case. But why is it NOT simultaneous? I could understand perhaps if it was, say, a sales tax; or VAT, something which may have only one effect (although I don't know if even this is true, u/Hou_Civil_Econ I believe is saying that here: https://www.reddit.com/r/AskEconomics/comments/qploog/why_does_taxation_not_shift_the_demand_curve/) but if that is the case why is it not the case that demand shifts left AND supply shifts left?

Answer 1

If I understand you correctly, your main question is why don't both demand and supply functions shift when the market is incurring a tax?

What I think makes this a bit confusing is the fact that the shifts of the demand or supply are just a way to more easily understand the concept graphically, as none of these shifts technically occur. A good starting point would be to define what shifts of the demand and supply curve actually mean. According to the textbook "Economics"(Begg et al) widely used in Introduction to Economics courses:

Shifts in demand curves happen when at least one of the following factors change:

1. Price of related goods
2. Consumer incomes
3. Tastes and expectations

Similarly, Shifts in supply curves happen when at least one of the following factors change:

1. Technology
2. Input costs
3. Goverment regulation (e.g. Environment regulations, this factor does not refer to tax)
4. Expectations

Since, when introducing tax in simple models like the one you mention, we assume ceteris paribus (all things equal), there is no reason to believe that either of these curves actually shift. Rather, the shifts are a means to more easily visualize the tax and get to the equations needed to calculate the new quantity demanded and suplied.

Since we are only moving along a supply or demand curve, consumers will reach point A on the graph, and suppliers will go to point B, and there will be an equilibrium because the quantity supplied and demanded are equal at Q. At this point:

$$p_b-p_s=t$$

And the tax incidence (amount of tax each party bears) will be, for consumers: $$i_b=p_b-p^*$$ and, for suppliers: $$i_s=p^*-p_s$$

I hope this clears things up a bit :)

Answer 2

I think you are correct. It could be both. It all depends on what you choose as a "price" in the representation of the inverse demand and supply curves.

Assume you have a demand function $D(p)$ and a supply function $S(p)$. If there is not tax, the equilibrium price $p^\ast$ is found when supply equals demand: $$ S(p^\ast) = D(p^\ast). $$ Now consider a tax of an amount $t$ (per unit). This tax makes it that the price the consumers pay (say $p^D$) is no longer the same as the one the producers receive (say $p^S$). In particular: $$ p^D = p^S + t. $$ Now, the new equilibrium still equates demand and supply: $$ D(p^D) = S(p^S). $$

If you want to present this in a price quantity setting, however, you need to decide on which price you put on the (say vertical) axis.

If you choose to represent everything in terms of $p^D$, you get the equation $$ D(p^D) = S(p^D - t). $$ This corresponds to a setting where the (inverse) supply curve shifts up by an amount $t$.

You could also choose to represent everything in terms of $p^S$. Then you get the equation: $$ D(p^S + t) = S(p^S). $$ This corresponds to shifting the (inverse) demand curve down by an amount $t$.

In fact, you could also opt to represent everything in terms of, say $\widetilde{p} = p^D - 0.5t = p^S + 0.5 t$. This would give: $$ D(\tilde p + 0.5t) = S(\tilde p - 0.5t). $$ This amounts to shifting the (inverse) supply curve up by an amount $0.5t$ and the (inverse) demand curve down by an amount equal to $0.5 t$

In my opinion, a great deal of difficulty related to these "shifts" comes from the fact that we tend to represent prices on the vertical axis and quantities on the horizontal axis. It would be better to do it the other way around as prices are the independent variable and quantity is the dependent one. If you put prices on the vertical axis and quantity on the horizontal one, you are in fact representing inverse demand and inverse supply functions. When you impose a tax, these are shifted up or down (not left or right).

For example after the tax, we have: $$ q^D = D(p^S + t). $$ Inverting this gives: $$ p^S = D^{-1}(q^D) - t, $$ So this amounts to a downward shift (by $t$) of the inverse demand function (if you represent prices in terms of $p^S$ on the vertical axis).