1
$\begingroup$

Question

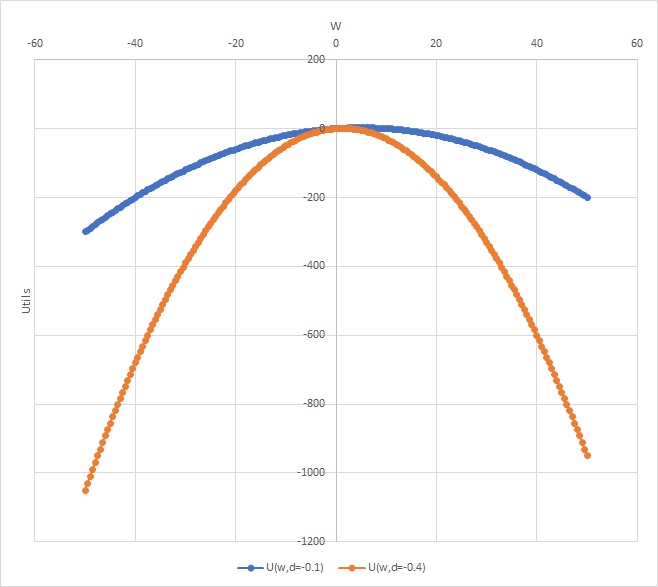
A risk-averse, non-satiated investor has decided to use the utility function $$U(w) = w + dw^2,$$ where $$d \leq 0$$ is a constant, to describe his preferences.

Derive an upper bound for $w$, in terms of $d$, for which $U(w)$ is valid.

My Answer

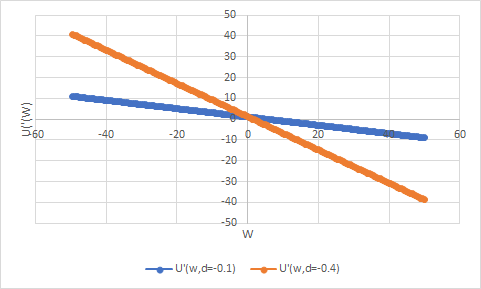
I know that, for non-satiation, $$U'(w) \geq 0.$$

Approach 1

$$\begin{aligned} 1 + 2dw \geq 0\\ \implies w \geq -\frac 1 {2d} \end{aligned}$$

Approach 2

Let $$e = -d.$$ $$\begin{aligned} 1 - 2ew \geq 0\\ \implies w \leq \frac 1 {2e}\\ \implies w \leq -\frac 1 {2d} \end{aligned}$$

Since the question is asking for an upper bound, I deduce that my second approach is the correct one. However, I would like to know why the first approach is incorrect.

Any intuitive explanations will be greatly appreciated :)

Improve this question
$\endgroup$
2
  • 2
    $\begingroup$ You need to track the sign of $d$ whether it's implicit (as in the first approach) or explicit (as in the second) when dividing. So even in the first, the inequality switches direction and you get the same result both ways. $\endgroup$
    – BrsG
    Commented Aug 2, 2022 at 14:48
  • $\begingroup$ Voting to close: The solution is unrelated to economics, but simply an application of rather basic math. Not worthwhile documenting here. $\endgroup$
    – BrsG
    Commented Aug 2, 2022 at 14:50

1 Answer 1

Reset to default
2
$\begingroup$

Note that your problem said to assume that $d < 0$. When you divide both sides of an inequality by a negative number, you flip the signs. For example: $$ -2x < 4 \Rightarrow x > -2 $$

Once you handle that, you can see that both methods imply the same relationship.

Levels: U(W)

First derivative: U'(W)

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.