According to this article USA owns $19 trillion-plus dollars.
So it seems USA owns $12 trillion to its own people. Why can't we just forgive it and remove this debt? People will not get this money anyway and whatever they get from the government is laughable. The economy and US currency would grow exponentially which would take care of the people's retirement funds.
Let's try the Fermi estimate.
In a two-period set up (each lasting something like 25 years in calendar time), let the intertemporal budget constraints be
$$a_2 =(1+r)a_1 + w_1 - c_1$$ $$c_2 = (1-b)a_2 +p_2, \;\;\; 0<b<1$$
where $a$ is assets, $w$ is wage income $c$ is consumption, $p$ is pension, and $b$ is bequest as a percentage of assets.
Assume that $a$ is i government bonds. Note that old people eat up their wealth : i.e. they collect back also the principal from the government (fully if $b=0$). This does not mean that government debt is extinguished, it just means that it changes hands.
Assume that the young person does not plan on making any more savings or dissavings, so $w_1 = c_1$, and it lets his current assets accumulate. Also assume that $b=0$: if we are talking about low and middle-income persons, this is not very unrealistic reality since we are talking strictly about assets in the form of government bonds.
Consider now wiping out the debt: it means that consumption when old will tend to go down by $a_2$. In order for the envisioned increased economic growth to "take care of pensions" we must have at least that the initial $p_2$ will increase by $a_2$.
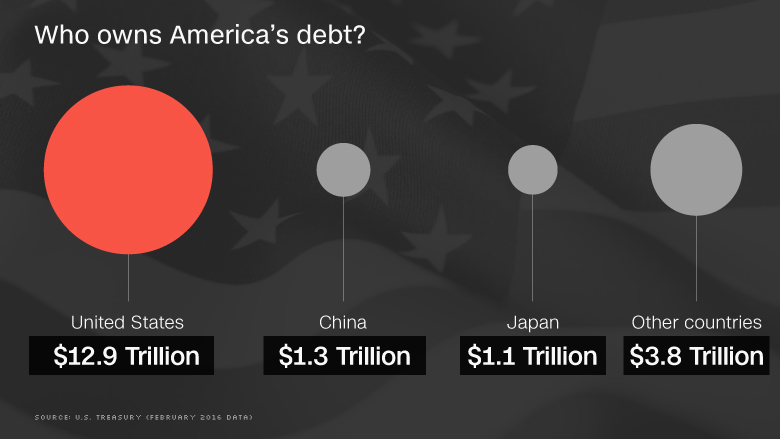
As calculated here, "nearly half of the debt is held in trust for retirement". Say $9$ trillion USD. Assume that the current real-interest rate on US bonds is $0.5$%. Compounded over $25$ years we get $1+r = 1.13$.
So if we wipe out the debt related to pensions, we must guarantee to the future old generation an additional $~10$ trillion USD in pensions (in real terms).
Current US GDP is $18$ trillion USD and it increases by $2.2$%. This will lead to $31$ trillion in $25$ years time. But we want that to increase by another $~10$ trillion at least, and assuming that all will be given for pensions. So we need GDP to reach in $25$ years $41$ trillion USD. For this to happen we need an average growth rate over the period not of $2.2$% but of $3.35$%. That's $1.15$ percentage points higher growth or more than $50$% higher average growth than today.
So our Fermi estimate led us to ask:
Can eliminating 9 trillion in Federal debt held for retirement purposes lead to an increase of the average growth for the next 25 years by $1.15$ percentage points?
Then in order to answer this question we must ask "how eliminating government debt will lead to increased growth" irrespective of how much.
I obviously ignore issues of uncertainty, property rights, and the like.
Most government debts in the world are in form of terms bonds. It is a sort of collateral base on trust. The fun parts about US debts, is that country that run GIGANTIC inficit trade against USA, can't avoid it buying US bonds.
Inbalance trade will cause the recipient side receive too much money, thus, will trigger inflation on them. To negate this effect, historically, countries like Japan and China will use surplus currency earn to buy USA bond to counter it.
Now, imagine the bonds is defaulted. With the lost of the trust on bonds, while the inbalanced trade still ongoing : countries with tsunami wave of earned money need to park the earned US dollar somewhere. Guess what happens when excessive amount of dollar (hundreds of billion every quarter) pour back to USA market? It will trigger inflation on almost everything, and the best tools (bonds) in the first place already destroyed.
I think the US has not yet defaulted on federal debt, so it seems people do get the money back, and hence the question is based on a false premise.
I see no reason why the economy would grow after debt forgiveness, unless you assume the debtors would be able to get new credit, which seems unlikely. After all it will just be forgiven again. As for debtors being able to spend money on consumption and investment rather than interest: This was still spent when debt existed, only not by the debtors, but by the creditors who got it.
On a side note: I like your attitude. Would you mind lending me money?
