Tit-For-Stat Strategy Best Replies

Question

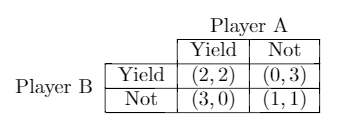
Let $\delta\in(0,1)$ be the discount factor. Consider the stage game in the infinitely repeated prisoner's dilemma game:

The goal is to derive conditions on $\delta$ such that the symmetric tit-for-tat strategy profile is a Nash equilibrium.

To recall, tit-for-tat is when a player cooperates (here plays Yield, $Y$) the first round and then every round after copies the action of their opponent the second round.

I am told that if A is playing tit-for-that then B's best possible replies would be to alternate between $N$ and $Y$ (playing $N$ first), to always play $N$, or to play tit-for-tat himself resulting in both players always playing $Y$.

We compute the expected payoffs of each depending on $\delta$ and then figure conditions on $\delta$ so that $B$ should play tit-for-tat. As the payoffs are symmetric, these conditions provide $A$ should also play tit-for-tat given $B$ is and we have a Nash.

My only question is why it must be that these are the only best possible replies. Why might not $B$ have some periods where he plays $N$ for a while then switches to only cooperating? Or vice versa. Or anything outside of these three.

Answer 1

Instead of calling the three strategies you named the "best possible replies", I would say that they are the most salient replies. As you mentioned, there are many other potential responses to a tit-for-tat strategy. It turns out that what best responds to a tit-for-tat strategy is also a tit-for-tat strategy. Every other strategy will produce a lower payoff than using the tit-for-tat.

Consider the sequence of outcomes generated by each of the three strategies you mentioned (played by $i$ against the other player's tit-for-tat):

• Tit-for-tat ($s_i^T$): $(Y,Y)$, $(Y,Y)$, $(Y,Y)$, $\dots$
• Alternating ($s_i^A$): $(Y,N)$, $(N,Y)$, $(Y,N)$, $\dots$
• Always $N$ ($s_i^N$): $(Y,N)$, $(N,N)$, $(N,N)$, $\dots$

Note that all four possible stage-game outcomes are observed. As a result, if a player $i$ is playing any other strategy $s_i^O$, her payoff $u_i(s_i^O,s_j^T)$ can be expressed as some convex combination of the payoffs from playing either of the above three strategies. That is, there exists $\alpha,\beta\in[0,1]$ with $\alpha+\beta\le1$ such that, for any $s_i^O\in S_i$, \begin{equation} u_i(s_i^O,s_j^T)=\alpha u_i(s_i^T,s_j^T) +\beta u_i(s_i^A,s_j^T) +(1-\alpha-\beta)u_i(s_i^N,s_j^T). \end{equation}

Now if you established that $u_i(s_i^T,s_j^T)\ge u_i(s_i^A,s_j^T)$ and $u_i(s_i^T,s_j^T)\ge u_i(s_i^N,s_j^T)$, which I assume you have done, it must follow that $u_i(s_i^T,s_j^T)\ge u_i(s_i^O,s_j^T)$ for any other strategy $s_i^O$.