2
$\begingroup$

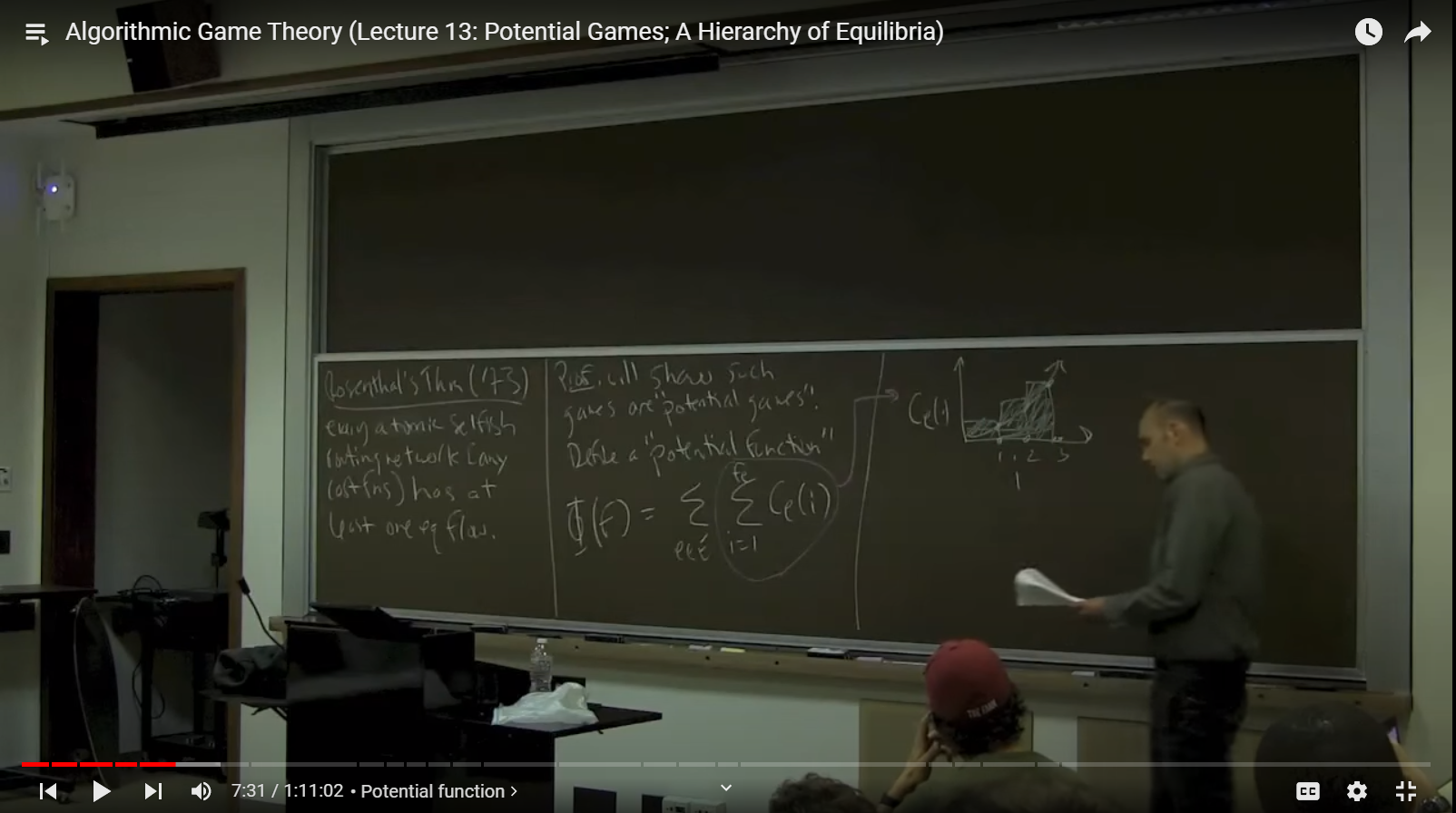
One geometric interpretation of (at least one term of) the potential function I've come across is as the Riemann-approximated area under an individual player's cost as a function of the number of players...

enter image description here

However, the potential function is not actually a function of the number of player, but of their strategies. Is there a geometric interpretation of the potential function for, i.e., a game with two players as a three-dimensional surface, where the input dimensions are the strategies of the two players or something like that, and the potential function's extrema (which correspond to Nash Equilibria) can be seen? Presumably, a game without a potential function would correspond to a surface without extrema?

Improve this question
$\endgroup$

1 Answer 1

Reset to default
2
$\begingroup$

If both players have a 1-dimensional strategy space, then the graph of the potential function is a "surface" over the 2-dimensional Cartesian product of these strategy spaces. This of course generalizes to higher dimensions, but then it can no longer be visualized for a geometric intuition.

However, a game without a potential function doesn't correspond to a "surface without extrema", it just corresponds to no such surface existing.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Is there a meaningful relationship between this notion of a potential function and the MV Calculus notion of a potential function (basically the inverse of the gradient operator), or is this an artificially constructed similarity? $\endgroup$
    – user10478
    Commented Jan 3, 2023 at 16:13
  • 1
    $\begingroup$ @user10478, as far as I remember there is some relationship that is more than just a similarity, but I don't know the details. Maybe try asking this question at Math StackExchange. $\endgroup$
    – VARulle
    Commented Jan 4, 2023 at 14:28

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.