Why does MRTS get smaller when there is labour saving technical change

Question

Context* both curves in the image have the same level of output, it shows the progress of technological change.

When labour efficiency has gotten proportionally better in comparison with capital, I would assume that less labour is needed to work a certain amount of capital to produce the same level of output. But on this graph when there is technological change moving from the blue graph to the red graph, if I were to choose a point of capital and looked at how much labour is needed on both curves to produce the same amount of output the red curve needs more labour to produce the same level of output at a fixed level of capital in comparison to the blue curve, so has it gotten better technologically labour wise?

Answer 1

You are correct.

---

Let's zoom out

When transitioning from the blue curve $q = L^{0.5}K^{0.5}$ to the red curve $q = L^{0.2}K^{0.5}$, the production function reflects labour saving technological change, where labour's contribution to output diminishes relative to capital. However, the effect depends on the level of capital $K$. Let's consider this region by region.

Note the chart below is not to scale.

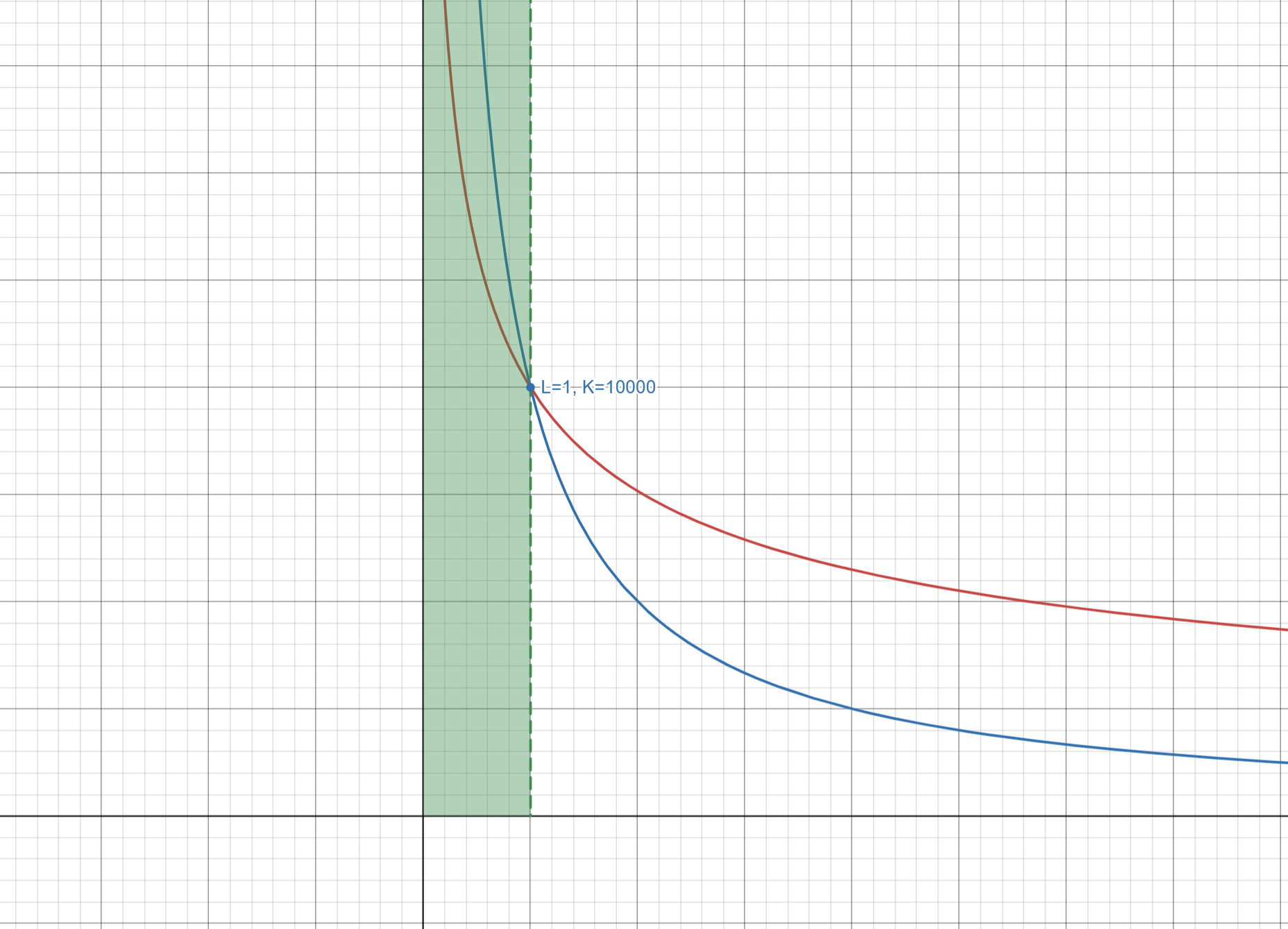
Low levels of capital $K < 10000$

At lower levels of $K$, capital is insufficient to compensate for the reduced role of labour in the red curve, where labour's productivity is weaker due to the smaller exponent $0.2$. Consequently, at fixed, low $K$, more labour is required on the red curve to achieve the same output as the blue curve:

$$ q = 100 \quad \implies\quad L_{\text{red}} > L_{\text{blue}}. $$

At $K = 10000$

The two curves intersect because the contribution of labour and capital balance out equally in both production functions. At this point, both curves require the same amount of labour to achieve the same output:

$$ L_{\text{red}} = L_{\text{blue}} = 1. $$

High levels of capital $K > 10000$

At higher levels of $K$, capital becomes abundant and can substitute more effectively for labour. This highlights the labour saving nature of the red curve, where less labour is required to achieve the same output compared to the blue curve:

$$ q = 100 \quad \implies\quad L_{\text{red}} < L_{\text{blue}}. $$

Takeaway

Labour saving technological change doesn't always reduce labor requirements at every capital level:

• For $K < 10000$, labour requirements may increase because labour's contribution to output is weaker in the red curve.
• For $K > 10000$, the benefits of labour saving technology become evident as capital substitutes for labour, reducing labour requirements.

Answer 2

When we think of technological progress, we think of the amount of measurable inputs, capital and labour, needed to produce a certain amount of output. As technology advances, you need less inputs to produce the same output.

For labour augmenting technological change in particular, for a fixed amount of $K$, the same amount of labour produces more output. As such, your graph cannot be representing labour augmenting technological change since the same amount produces less output.

Mathematically, we tend to represent this as

$$Y_t = F(K_t,A^L_t L_t).$$

As a result the MRTS is

$$MRS^{L}_{Kt} = \frac{\frac{\partial Y_{t}}{\partial L_t}}{\frac{\partial Y_{t}}{\partial K_t}} = \frac{F_2}{F_1} A^{L}_t $$

where $F_i$ is the partial derivative of function $F(\cdot)$ wrt term $i$. Note that, fixing $L_t$ and $K_t$, as $A^L_t$ increases, the MRTS increases, as your intuition rightly pointed out, meaning that we need more capital to replace the same amount of labour.

Hope this helps.