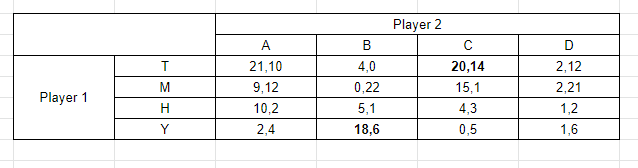
I would like to find the set of rationalizable strategies for this 4x4 game:
The first thing I did was try and find all PSNE. I found two, the ones I bolded.
Thus, my answer to this question is that the set of rationalizable strategies for Player 1 is {T, Y} and the set of rationalizable strategies for Player 2 is {B, C}.
Is this answer correct?